Nota
Fare clic qui per scaricare il codice di esempio completo
Annotazione dei grafici #
I seguenti esempi mostrano come è possibile annotare grafici in Matplotlib. Ciò include l'evidenziazione di punti di interesse specifici e l'utilizzo di vari strumenti visivi per richiamare l'attenzione su questo punto. Per una descrizione più completa e approfondita degli strumenti di annotazione e testo in Matplotlib, vedere il tutorial sull'annotazione .
import matplotlib.pyplot as plt
from matplotlib.patches import Ellipse
import numpy as np
from matplotlib.text import OffsetFrom
Specificare punti di testo e punti di annotazione #
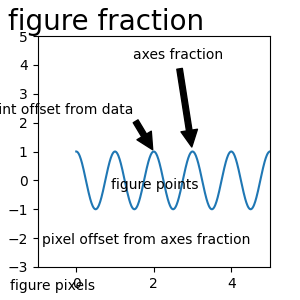
È necessario specificare un punto di annotazione per annotare questo punto. Inoltre, puoi specificare un punto di testo per la posizione del testo per questa annotazione. Facoltativamente, puoi specificare il sistema di coordinate di xy e xytext con una delle seguenti stringhe per xycoords
e textcoords (il valore predefinito è 'data'):xy=(x, y)xytext=(x, y)
'figure points' : points from the lower left corner of the figure
'figure pixels' : pixels from the lower left corner of the figure
'figure fraction' : (0, 0) is lower left of figure and (1, 1) is upper right
'axes points' : points from lower left corner of axes
'axes pixels' : pixels from lower left corner of axes
'axes fraction' : (0, 0) is lower left of axes and (1, 1) is upper right
'offset points' : Specify an offset (in points) from the xy value
'offset pixels' : Specify an offset (in pixels) from the xy value
'data' : use the axes data coordinate system
Nota: per i sistemi di coordinate fisiche (punti o pixel) l'origine è (in basso, a sinistra) della figura o degli assi.
Facoltativamente, puoi specificare le proprietà della freccia che disegna una freccia dal testo al punto annotato fornendo un dizionario delle proprietà della freccia
Le chiavi valide sono:
width : the width of the arrow in points
frac : the fraction of the arrow length occupied by the head
headwidth : the width of the base of the arrow head in points
shrink : move the tip and base some percent away from the
annotated point and text
any key for matplotlib.patches.polygon (e.g., facecolor)
# Create our figure and data we'll use for plotting
fig, ax = plt.subplots(figsize=(3, 3))
t = np.arange(0.0, 5.0, 0.01)
s = np.cos(2*np.pi*t)
# Plot a line and add some simple annotations
line, = ax.plot(t, s)
ax.annotate('figure pixels',
xy=(10, 10), xycoords='figure pixels')
ax.annotate('figure points',
xy=(80, 80), xycoords='figure points')
ax.annotate('figure fraction',
xy=(.025, .975), xycoords='figure fraction',
horizontalalignment='left', verticalalignment='top',
fontsize=20)
# The following examples show off how these arrows are drawn.
ax.annotate('point offset from data',
xy=(2, 1), xycoords='data',
xytext=(-15, 25), textcoords='offset points',
arrowprops=dict(facecolor='black', shrink=0.05),
horizontalalignment='right', verticalalignment='bottom')
ax.annotate('axes fraction',
xy=(3, 1), xycoords='data',
xytext=(0.8, 0.95), textcoords='axes fraction',
arrowprops=dict(facecolor='black', shrink=0.05),
horizontalalignment='right', verticalalignment='top')
# You may also use negative points or pixels to specify from (right, top).
# E.g., (-10, 10) is 10 points to the left of the right side of the axes and 10
# points above the bottom
ax.annotate('pixel offset from axes fraction',
xy=(1, 0), xycoords='axes fraction',
xytext=(-20, 20), textcoords='offset pixels',
horizontalalignment='right',
verticalalignment='bottom')
ax.set(xlim=(-1, 5), ylim=(-3, 5))
[(-1.0, 5.0), (-3.0, 5.0)]
Utilizzo di più sistemi di coordinate e tipi di assi #
È possibile specificare il punto xy e il testo xy in diverse posizioni e sistemi di coordinate e, facoltativamente, attivare una linea di collegamento e contrassegnare il punto con un indicatore. Le annotazioni funzionano anche sugli assi polari.
Nell'esempio seguente, il punto xy si trova nelle coordinate native ( xycoords è predefinito su 'data'). Per un asse polare, questo è nello spazio (theta, raggio). Il testo nell'esempio viene inserito nel sistema di coordinate della figura frazionaria. Gli argomenti delle parole chiave del testo come l'allineamento orizzontale e verticale vengono rispettati.
fig, ax = plt.subplots(subplot_kw=dict(projection='polar'), figsize=(3, 3))
r = np.arange(0, 1, 0.001)
theta = 2*2*np.pi*r
line, = ax.plot(theta, r)
ind = 800
thisr, thistheta = r[ind], theta[ind]
ax.plot([thistheta], [thisr], 'o')
ax.annotate('a polar annotation',
xy=(thistheta, thisr), # theta, radius
xytext=(0.05, 0.05), # fraction, fraction
textcoords='figure fraction',
arrowprops=dict(facecolor='black', shrink=0.05),
horizontalalignment='left',
verticalalignment='bottom')

Text(0.05, 0.05, 'a polar annotation')
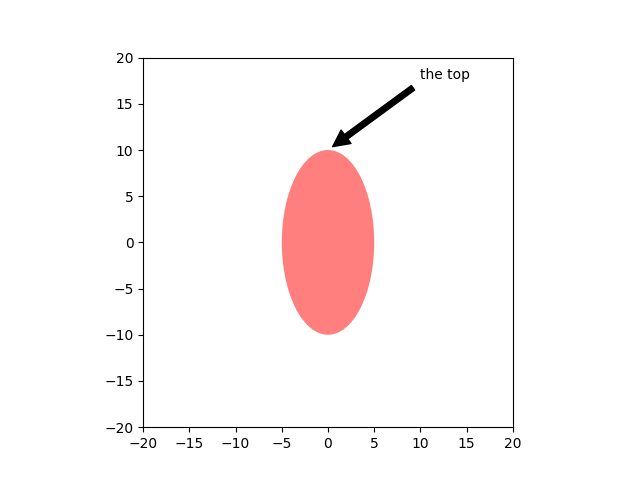
Puoi anche usare la notazione polare su un asse cartesiano. Qui il sistema di coordinate nativo ("data") è cartesiano, quindi è necessario specificare xycoords e textcoords come "polar" se si desidera utilizzare (theta, radius).
el = Ellipse((0, 0), 10, 20, facecolor='r', alpha=0.5)
fig, ax = plt.subplots(subplot_kw=dict(aspect='equal'))
ax.add_artist(el)
el.set_clip_box(ax.bbox)
ax.annotate('the top',
xy=(np.pi/2., 10.), # theta, radius
xytext=(np.pi/3, 20.), # theta, radius
xycoords='polar',
textcoords='polar',
arrowprops=dict(facecolor='black', shrink=0.05),
horizontalalignment='left',
verticalalignment='bottom',
clip_on=True) # clip to the axes bounding box
ax.set(xlim=[-20, 20], ylim=[-20, 20])
[(-20.0, 20.0), (-20.0, 20.0)]
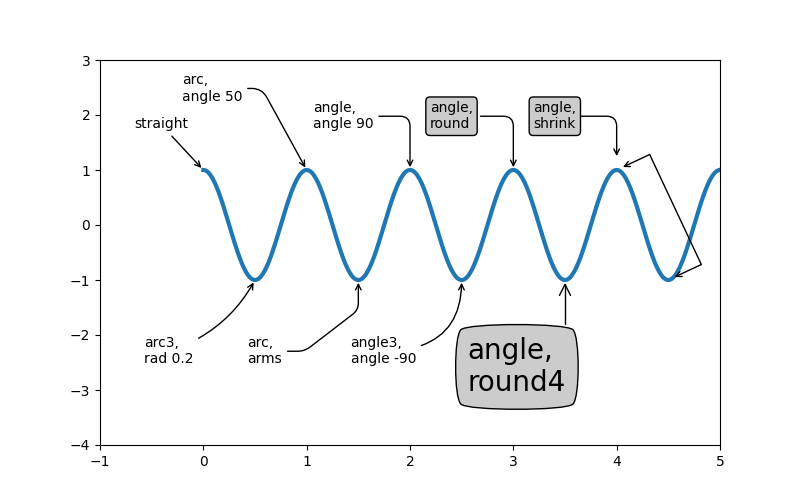
Personalizzazione degli stili di frecce e bolle #
La freccia tra xytext e il punto di annotazione, così come la bolla che copre il testo dell'annotazione, sono altamente personalizzabili. Di seguito sono riportate alcune opzioni di parametro e l'output risultante.
fig, ax = plt.subplots(figsize=(8, 5))
t = np.arange(0.0, 5.0, 0.01)
s = np.cos(2*np.pi*t)
line, = ax.plot(t, s, lw=3)
ax.annotate(
'straight',
xy=(0, 1), xycoords='data',
xytext=(-50, 30), textcoords='offset points',
arrowprops=dict(arrowstyle="->"))
ax.annotate(
'arc3,\nrad 0.2',
xy=(0.5, -1), xycoords='data',
xytext=(-80, -60), textcoords='offset points',
arrowprops=dict(arrowstyle="->",
connectionstyle="arc3,rad=.2"))
ax.annotate(
'arc,\nangle 50',
xy=(1., 1), xycoords='data',
xytext=(-90, 50), textcoords='offset points',
arrowprops=dict(arrowstyle="->",
connectionstyle="arc,angleA=0,armA=50,rad=10"))
ax.annotate(
'arc,\narms',
xy=(1.5, -1), xycoords='data',
xytext=(-80, -60), textcoords='offset points',
arrowprops=dict(
arrowstyle="->",
connectionstyle="arc,angleA=0,armA=40,angleB=-90,armB=30,rad=7"))
ax.annotate(
'angle,\nangle 90',
xy=(2., 1), xycoords='data',
xytext=(-70, 30), textcoords='offset points',
arrowprops=dict(arrowstyle="->",
connectionstyle="angle,angleA=0,angleB=90,rad=10"))
ax.annotate(
'angle3,\nangle -90',
xy=(2.5, -1), xycoords='data',
xytext=(-80, -60), textcoords='offset points',
arrowprops=dict(arrowstyle="->",
connectionstyle="angle3,angleA=0,angleB=-90"))
ax.annotate(
'angle,\nround',
xy=(3., 1), xycoords='data',
xytext=(-60, 30), textcoords='offset points',
bbox=dict(boxstyle="round", fc="0.8"),
arrowprops=dict(arrowstyle="->",
connectionstyle="angle,angleA=0,angleB=90,rad=10"))
ax.annotate(
'angle,\nround4',
xy=(3.5, -1), xycoords='data',
xytext=(-70, -80), textcoords='offset points',
size=20,
bbox=dict(boxstyle="round4,pad=.5", fc="0.8"),
arrowprops=dict(arrowstyle="->",
connectionstyle="angle,angleA=0,angleB=-90,rad=10"))
ax.annotate(
'angle,\nshrink',
xy=(4., 1), xycoords='data',
xytext=(-60, 30), textcoords='offset points',
bbox=dict(boxstyle="round", fc="0.8"),
arrowprops=dict(arrowstyle="->",
shrinkA=0, shrinkB=10,
connectionstyle="angle,angleA=0,angleB=90,rad=10"))
# You can pass an empty string to get only annotation arrows rendered
ax.annotate('', xy=(4., 1.), xycoords='data',
xytext=(4.5, -1), textcoords='data',
arrowprops=dict(arrowstyle="<->",
connectionstyle="bar",
ec="k",
shrinkA=5, shrinkB=5))
ax.set(xlim=(-1, 5), ylim=(-4, 3))
[(-1.0, 5.0), (-4.0, 3.0)]
Creeremo un'altra figura in modo che non sia troppo ingombra
fig, ax = plt.subplots()
el = Ellipse((2, -1), 0.5, 0.5)
ax.add_patch(el)
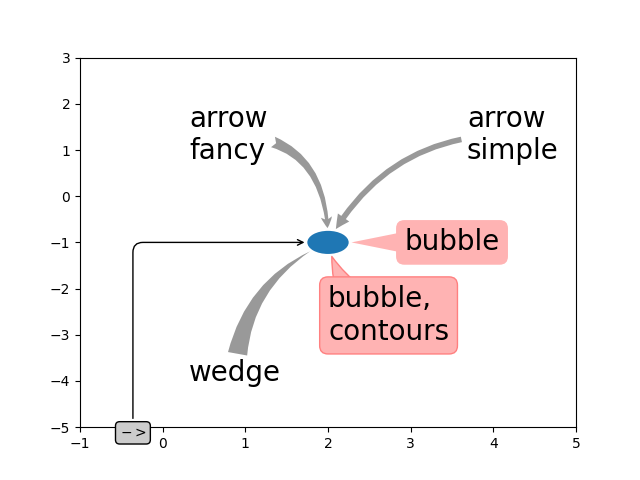
ax.annotate('$->$',
xy=(2., -1), xycoords='data',
xytext=(-150, -140), textcoords='offset points',
bbox=dict(boxstyle="round", fc="0.8"),
arrowprops=dict(arrowstyle="->",
patchB=el,
connectionstyle="angle,angleA=90,angleB=0,rad=10"))
ax.annotate('arrow\nfancy',
xy=(2., -1), xycoords='data',
xytext=(-100, 60), textcoords='offset points',
size=20,
arrowprops=dict(arrowstyle="fancy",
fc="0.6", ec="none",
patchB=el,
connectionstyle="angle3,angleA=0,angleB=-90"))
ax.annotate('arrow\nsimple',
xy=(2., -1), xycoords='data',
xytext=(100, 60), textcoords='offset points',
size=20,
arrowprops=dict(arrowstyle="simple",
fc="0.6", ec="none",
patchB=el,
connectionstyle="arc3,rad=0.3"))
ax.annotate('wedge',
xy=(2., -1), xycoords='data',
xytext=(-100, -100), textcoords='offset points',
size=20,
arrowprops=dict(arrowstyle="wedge,tail_width=0.7",
fc="0.6", ec="none",
patchB=el,
connectionstyle="arc3,rad=-0.3"))
ax.annotate('bubble,\ncontours',
xy=(2., -1), xycoords='data',
xytext=(0, -70), textcoords='offset points',
size=20,
bbox=dict(boxstyle="round",
fc=(1.0, 0.7, 0.7),
ec=(1., .5, .5)),
arrowprops=dict(arrowstyle="wedge,tail_width=1.",
fc=(1.0, 0.7, 0.7), ec=(1., .5, .5),
patchA=None,
patchB=el,
relpos=(0.2, 0.8),
connectionstyle="arc3,rad=-0.1"))
ax.annotate('bubble',
xy=(2., -1), xycoords='data',
xytext=(55, 0), textcoords='offset points',
size=20, va="center",
bbox=dict(boxstyle="round", fc=(1.0, 0.7, 0.7), ec="none"),
arrowprops=dict(arrowstyle="wedge,tail_width=1.",
fc=(1.0, 0.7, 0.7), ec="none",
patchA=None,
patchB=el,
relpos=(0.2, 0.5)))
ax.set(xlim=(-1, 5), ylim=(-5, 3))
[(-1.0, 5.0), (-5.0, 3.0)]
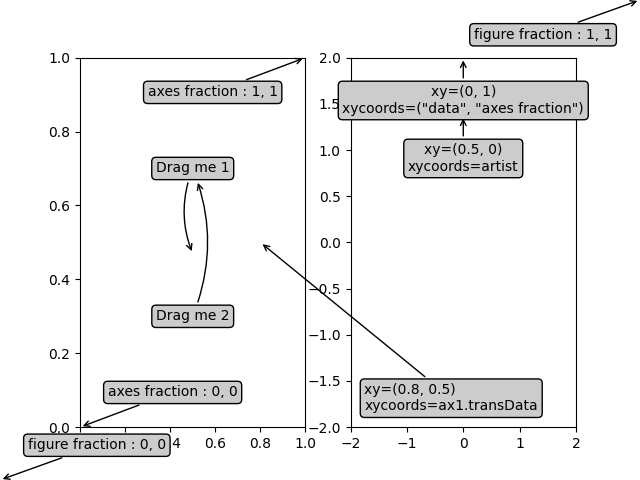
Altri esempi di sistemi di coordinate #
Di seguito mostreremo alcuni altri esempi di sistemi di coordinate e come specificare la posizione delle annotazioni.
fig, (ax1, ax2) = plt.subplots(1, 2)
bbox_args = dict(boxstyle="round", fc="0.8")
arrow_args = dict(arrowstyle="->")
# Here we'll demonstrate the extents of the coordinate system and how
# we place annotating text.
ax1.annotate('figure fraction : 0, 0', xy=(0, 0), xycoords='figure fraction',
xytext=(20, 20), textcoords='offset points',
ha="left", va="bottom",
bbox=bbox_args,
arrowprops=arrow_args)
ax1.annotate('figure fraction : 1, 1', xy=(1, 1), xycoords='figure fraction',
xytext=(-20, -20), textcoords='offset points',
ha="right", va="top",
bbox=bbox_args,
arrowprops=arrow_args)
ax1.annotate('axes fraction : 0, 0', xy=(0, 0), xycoords='axes fraction',
xytext=(20, 20), textcoords='offset points',
ha="left", va="bottom",
bbox=bbox_args,
arrowprops=arrow_args)
ax1.annotate('axes fraction : 1, 1', xy=(1, 1), xycoords='axes fraction',
xytext=(-20, -20), textcoords='offset points',
ha="right", va="top",
bbox=bbox_args,
arrowprops=arrow_args)
# It is also possible to generate draggable annotations
an1 = ax1.annotate('Drag me 1', xy=(.5, .7), xycoords='data',
ha="center", va="center",
bbox=bbox_args)
an2 = ax1.annotate('Drag me 2', xy=(.5, .5), xycoords=an1,
xytext=(.5, .3), textcoords='axes fraction',
ha="center", va="center",
bbox=bbox_args,
arrowprops=dict(patchB=an1.get_bbox_patch(),
connectionstyle="arc3,rad=0.2",
**arrow_args))
an1.draggable()
an2.draggable()
an3 = ax1.annotate('', xy=(.5, .5), xycoords=an2,
xytext=(.5, .5), textcoords=an1,
ha="center", va="center",
bbox=bbox_args,
arrowprops=dict(patchA=an1.get_bbox_patch(),
patchB=an2.get_bbox_patch(),
connectionstyle="arc3,rad=0.2",
**arrow_args))
# Finally we'll show off some more complex annotation and placement
text = ax2.annotate('xy=(0, 1)\nxycoords=("data", "axes fraction")',
xy=(0, 1), xycoords=("data", 'axes fraction'),
xytext=(0, -20), textcoords='offset points',
ha="center", va="top",
bbox=bbox_args,
arrowprops=arrow_args)
ax2.annotate('xy=(0.5, 0)\nxycoords=artist',
xy=(0.5, 0.), xycoords=text,
xytext=(0, -20), textcoords='offset points',
ha="center", va="top",
bbox=bbox_args,
arrowprops=arrow_args)
ax2.annotate('xy=(0.8, 0.5)\nxycoords=ax1.transData',
xy=(0.8, 0.5), xycoords=ax1.transData,
xytext=(10, 10),
textcoords=OffsetFrom(ax2.bbox, (0, 0), "points"),
ha="left", va="bottom",
bbox=bbox_args,
arrowprops=arrow_args)
ax2.set(xlim=[-2, 2], ylim=[-2, 2])
plt.show()
Tempo di esecuzione totale dello script: (0 minuti 2,463 secondi)